問1
(i)の答 下図1, 2 および 3
ここで, 1〜16 は Na+ を表わし, 図2と3の薄い緑色は海のような非極在化された電子を意味し, その電子は全てのナトリウムイオンの間に分配されそれらと一緒に一種の糊のような結合として作用する。
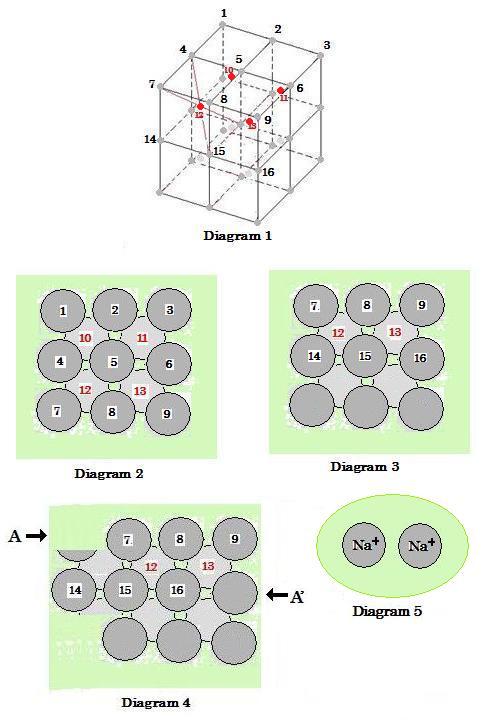
Diagram 1 : Na結晶の格子モデル (Na点と結合棒によるモデル, 1つの単位セルはb.c.c.である)。
Diagram 2 : Diagram 1中のNa結晶のトップ図()。
Diagram 3 : Diagram 1中のNa結晶の側面図。
Diagram 4 : Diagram 1中のNa結晶の側面図で, そのNa+層が2つの力 AとA’を加えることによって全体の構造を破壊することなく相互に滑っている。
Diagram 5 : Na2 分子。
次のことを注意, すなわち Diagram 2〜5 の薄い緑色は非極在化電子を表わしその電子は全てのナトリウムイオンに分配され結合されている。
● 金属原子が固体で相互に接近すると, 各原子は 1個以上の価電子において制御を失う。この電子はもはや特定の金属原子とは結びつかないで金属片を通して自由に動く : その電子は非極在化されているという。
いま非極在化されている価電子の存在で, 金属原子は事実上イオン化される。例えば, ナトリウム原子の電子構造は Na 1s22s22p63s1である。2つの内側の殻は満たされている。3s電子が非極在化され, 各ナトリウム原子をナトリウムイオンとして残る (電子構造 Na+ 1s22s22p6) :
Na 1s22s22p63s1 → (ionized) → Na+ 1s22s22p6
● その結合力は正のナトリウムイオンと非極在化された電子との間の引力である。非極在化電子は全てのナトリウムイオン間に分配され共に一種の’糊’のような結合として行動する。
● イオンと電子の全システムはイオンがそれ自身対称形に配列するとき最小エネルギーを持つ。それ故, 結合している固体金属は金属イオンの規則的配列から成り (格子と呼ばれている), ’海’のような非極在化された電子によって取り囲まれている。ナトリウム金属の場合では, その対称形は体心立方(b.c.c.)として記述される。
Q1
Answer of (i)
Diagrams 1, 2 and 3 below,
where 1〜16 show Na+s, and the light green color in diagrams 2 and 3 means a 'sea' delocalized electrons, that are shared between all the sodium ions and act as a sort of 'glue' bonding them together.
 (image556)
(image556)Diagram 1 : Lattice model of Na crystal (Na point-and-bond stick model. An unit cell is b.c.c.).
Diagram 2 : Top view of Na crystal in the diagram 1.
Diagram 3 : Side view of Na crystal in the diagram 1.
Diagram 4 : Side view of Na crystal in the diagram 1: the Na+ layers of which slip past one another without breaking on the whole structure by adding two forces A and A'.
Diagram 5 : Na2 molecule.
Note that the light green colour in diagrams 2〜5 is delocalized electrons which bond all the sodium ions.
● When metal atoms are close to each other in the solid, each atom loses control over one or more of its valence electrons. These electrons are no longer associated with a particular metal atom but are free to move throughout the solid piece of metal : the electrons are said to be delocalized.
With valence electrons now delocalized, the metal atoms are effectively ionized. For example, the electronic structure of a sodium atom is Na 1s22s22p63s1. The two inner shells are full. The 3s electron is delocalized, leaving each sodium atom as the sodium ion (electronic structure Na+ 1s22s22p6) :
Na 1s22s22p63s1 → (ionized) → Na+ 1s22s22p6
● The bonding force is the attraction between the positive sodium ions and the delocalized electrons. The delocalized electrons are shared between all the sodium ions and act as a sort of 'glue' holding them together.
● The whole system of ions and electrons has the lowest energy when the ions arrange themselves in a symmetrical pattern. Solid metallic bonding therefore consists of regular arrangements of metal ions (called a lattice), surrounded by a 'sea' of delocalized electrons. In the case of sodium metal, the symmetrical pattern is described as body-centred cubic (b.c.c.).
(ii)の答
上図4に示されるように, 金属は2つの力 AとA'を加えることによって形が崩されるとき, 金属イオンの層が全体の構造を壊すことなく相互に滑り通すことができる。ナトリウム固体のような金属はそれ故普通たたき続けたり圧力を加えたりすると新しい形になり, それは展性と呼ばれ, また, 引き延ばすとワイヤーになり, それは延性と呼ばれる。
● 金属は普通展性の性質を持つ : 展性物質はたたき続けたり圧力を加えると新しい形になる。また延性の性質を持つ : 延性物質はワイヤーに引き伸ばされ引き延ばすことによってより薄くなる。
金属が形を崩すとき, 固体構造中の金属イオンは全構造を破壊することなく相互に滑り通しそれに連れて試料全体の形は変化する。固体構造中のイオンと非極在化された電子の内部配列はこの滑りを許し結合力の顕著な破壊なしに行われる。
● 上図4において, Na金属が2つの力AとA'を加えることによって変形するとき, 金属イオンの層が全体の構造を破壊することなく相互に滑り通すことができる。
Answer of (ii)
As shown in the diagram 4 above, when a metal is deformed by adding two forces A and A', the layers of the metal ions can slip past one another without breaking up the whole structure. A metal such as sodium solid is therefore usually beaten or pressed into new shapes, called 'malleability', and also drawn out into a wire, called 'ductility'.
● Metals have usually the property of malleability : a malleable material may be beaten or pressed into new shapes. They have also usually the property of ductility : a ductile material may be drawn out into a wire and made thinner by stretching.
When metals are deformed, the metal ions in the solid structure slide past each other without breaking up the whole structure as the overall shape of the sample changes. The internal arrangement of ions and delocalized electrons in the solid structure allows this slippage to take place without significant disruption of the bonding forces.
● In the diagram 4 above, when the Na metal is deformed by adding two forces A and A, layers of the metal ions can slip past one another without breaking up the whole structure.
問2の答
マススペクトルの軌跡とナトリウムの相対質量が23.0であることから, ナトリウム蒸気分子の式はNa2として決定され, それは一対の非極在化された電子を持つ。かくしてその結合を示す図は上のDiagram 5に対応する。
上の軌跡でのナトリウム蒸気のマススペクトルは46のm/eでのみ高い強度を示している。これはナトリウム蒸気粒子の相対質量は46であることを意味している。このマススペクトルの情報とNaの相対原子量が23.0であることから, モルでのナトリウム蒸気粒子の量は次のように計算される :
46/23 = 2.
それゆえ, ナトリウム蒸気分子の式はNa2として決定され, それは一対の非極在化された電子をもつ。かくしてその結合を示す図は上のDiagram 5に対応する。
Answer of Q2
From the mass spectrum trace and the relative atomic mass of Na is 23.0, the formula of sodium vapor molecule is therefore determined as Na2 which has one pair of delocalized electrons. Thus a diagram showing the bonding is correspond to Diagram 5 above.
The mass spectrum for sodium vapor in the trace above indicates the high intensity only at 46 of m/e. This means that the relative mass of a sodium vapor particle is 46. From this mass spectrum information and the relative atomic mass of Na is 23.0, the amount of the sodium vapor particle in moles is calculated as follows :
46/23 = 2.
The formula of sodium vapor molecule is therefore determined as Na2, with one pair of delocalized shared electrons. Thus a diagram showing the bonding is corresponded to Diagram 5 above.
問3
(i)の答 2.4×10-5 g mol-1 (有効数字2桁)
理想気体の気体方程式は
PV = (W/M)RT
である。ここで,
P = 25 Nm-2,
V = 50 cm3 = 5.0×10-5 m3,
M = 46 g mol-1,
R = 8.31 J mol-1K-1 = 8.31 Nm mol-1K-1,
そして
T = 20 ℃ = 293 K.
そこで街灯中のナトリウム蒸気の質量 W (g) は, 上のPからTの各値を気体の方程式に代入し, 計算されると
W = MPV/RT
= (46 g mol-1)(25 Nm-2)(5.0×10-5 m3)/{(8.31 Nm mol-1K-1)(293 K)}
= 2.4×10-5 g (有効数字2桁)
Q3
Answer of (i) 2.4×10-5 g mol-1 (2 sig. figs)
The equation of ideal gas is
PV = (W/M)RT,
where
P = 25 Nm-2,
V = 50 cm3 = 5.0×10-5 m3,
M = 46 g mol-1,
R = 8.31 J mol-1K-1 = 8.31 Nm mol-1K-1,
and
T = 20 ℃ = 293 K.
Substrate each of the values above for the equation of ideal gas to calculate the mass of sodium in a street lamp, W (g), and so
W = MPV/RT
= (46 g mol-1)(25 Nm-2)(5.0×10-5 m3)/{(8.31 Nm mol-1K-1)(293 K)}
= 2.4×10-5 g (2 sig. figs)
(ii)の答
重要な仮定としてナトリウム気体の分子は : 絶えずランダムな動きをしており, 分子間力が無く, 完全弾性衝突で, 体積がない。
Answer of (ii)
It is important assumption that the molecules of sodium gas : are in continuous random motion, experience no intermolecular forces, have perfectly elastic collisions, and occupy zero volume.
問4
(i)の答 Na 1s22s22p63s1
Q4
Answer of (i) Na 1s22s22p63s1
(ii)の答
ナトリウム原子での 1s から 3s までの相対エネルギーは 1s < 2s < 2p < 3s の順で増加する。最外殻の3sの電子は最も高いエネルギーをもっているので, 一価の電荷 1+ のナトリウム陽イオンがそのもとの原子から3s電子を失うことで容易に形成される。
Answer of (ii)
The relative energies of the 1s to 3s atomic orbital for sodium atom increase in the order 1s < 2s < 2p < 3s. One electron of 3s in the most outer orbital has the highest energy, so the positive sodium ion with a charge 1+ is easily formed by the loss of the 3s electron from its parent atom.
(iii)の答 Na* 1s22s22p63p1
Answer of (iii) Na* 1s22s22p63p1